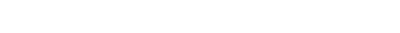Abstract

This article centers on the acoustical principles involved in forming a new set of steel-keyed American gamelan instruments referred to as the “Lightbulb Instruments,” and it describes the approach behind building and tuning the instruments. These steel “gamelan” instruments, built by Brian Baumbusch in collaboration with American sculptor Bernie Hosey, are based on a five-tone scale evocative of a subset of the Indonesian pelog scale, but they are tailored to be self-generative in that the tuning of each key is extracted from an overtone from a lower pitched key. William Setharis outlines this theory of tuning in his book Tuning, Timbre, Spectrum, Scale, and Baumbusch’s acoustical approach draws significant inspiration from this work. Similarly, this new approach to acoustic theory derives influence from the work of James Tenney as well as the temperament theory of Erv Wilson, but it departs significantly from past scholarship by Hermann Helmholtz and Harry Partch.

A New Model for American Gamelan Tuning

In this article, I briefly describe a tuning model used to create a new set of American gamelan instruments. To begin, here is a glossary of terms specific to the realm of acoustics that I discuss in the hopes that it will be informative and will provide a soft introduction to the subject material. In certain instances, these definitions are provided to illustrate the distinction in the way I will use these terms, which may differ from some of their common contemporary usages.

Scale

A stepwise arrangement of pitches with specific interval sizes in relation to the starting pitch (and with each other) that repeat roughly at the octave.

Key

An individual metal bar, groups of which make up full metallophones. This is not to be confused with a pitch center or a key signature.

2/1 Octave (true octave)

An interval with an exact pitch relationship of 2/1 (for example, 440hz/220hz).

Frequency

The rate at which the pressure created through a sound wave oscillates. (In this article, this is interchangeable with pitch.)

Fundamental Frequency

The lowest frequency created by a vibrating body. In metal bars, this is produced by the vibration of the first transverse mode. In harmonic instruments, it is produced by the first harmonic.

Unison

Any number of pitches located at the same scale degree with the same approximate frequency (within the critical band), although not necessarily identical. (Here, unison describes paired pitches with close frequencies that produce different frequency beats.

Amplitude

Volume, dynamic intensity.

X ̅

The average frequency taken from a group of frequencies.

Iº, I’, I’’

Iº = Scale degree I, 8vb (one octave below)

I’ = Scale degree I, 8va (one octave above)

I’’ = Scale degree I, 15ma (two octaves above)

Overtones

Tones higher in pitch than the fundamental frequency produced by partial vibrations (partials) of a sounding body.

Mode(s)

In physics and engineering, resonant modes are defined as the different manners of vibration throughout a vibrating body that constitute its full vibrating energy. These can also be considered as related to overtones, where modes are aspects of physical vibration and overtones are the resultant frequencies. Types of resonant modes in metal bars include the transverse modes, torsional modes, lateral modes, etc.

Spectrum

The total content of overtones of a sounding body, the composite of which represents the full vibrational energy of the sound. Human perception of the timbre of sounds is largely determined by spectrum and its change over time.

Node

A physical point in a mode of vibration where no vibration occurs. Its opposite is an antinode, which is the mode’s point of maximal vibration.

Inharmonic

The manner of vibration of most idiophones and membranophones, which is different from harmonic vibration in that the partials do not correspond with ascending integer relationships, a.k.a. the harmonic series, as they more closely do in harmonic instruments, such as chordophones and aerophones. The degree of inharmonicity can also be considered the degree to which vibrations depart from the harmonic series.

Ombak

The Balinese term for a difference in frequency beat, which occurs when two pitches are close enough together that they combine to form a secondary wave with regularly recurring amplitude modulations, like a tremolo.

Pengisup

The Balinese term for the higher pitched instrument within a pair.

Pengumbang

The Balinese term for the lower pitched instrument within a pair.

Alto+, Soprano+, Tenor+

The higher-pitched instrument in a pair of metallophones, usually at unison scale degrees (equivalent to pengisup).

Alto-, Soprano-, Tenor-

The lower-pitched instrument in a pair of metallophones (equivalent to pengumbang).

The term “American gamelan,” although somewhat amorphous in its application, describes a cultural phenomenon that has evolved over the past century and now applies to a range of practices both in the performing arts and in the academic field. The instruments I describe can be included as part of that cultural phenomenon and are as much a product of American experimentalism as they are of Indonesian gamelan music. These instruments, although heavily inspired by Balinese gamelan instruments, also depart from some of the standardized practices in Indonesian instrument building as well as Western tuning theory and acoustics. With these departures, I have incorporated a few theories of contemporary acoustician William Sethares, and I have reinterpreted some antecedent theories by Hermann Helmholtz and Harry Partch. To elaborate on my building practices, I describe both the rough-tuning and the fine-tuning process involved in making the keys of the instruments, and I show how models of symmetry and inharmonicity were used in tandem with established tuning practices from Bali to create a base-scale and an inter-key relationship unique to my own partiality.

A Brief Background

American gamelan music is in no way a new cultural phenomenon. In the past fifty years and in many instrument-building endeavors, Americans have drawn inspiration from Indonesian gamelan instruments to build their own unique sets of instruments on which to perform their own music. Predating those endeavors, an established cultural interchange between Indonesian gamelan music and Western concert music persisted throughout the twentieth century. Early proponents of that interchange were German painter Walter Spies (1895–1942), who drew international attention to Balinese music in the early part of the twentieth century, and Canadian composer Colin McPhee (1900–1964), who composed large-scale orchestral works and other concert music that was overtly influenced by and drew direct musical quotes from Balinese gamelan music. (Other well-known composers, including Claude Debussy [1862–1918] and Percy Grainger [1900–1961], were influenced by gamelan music in their compositional life.) Another important figure in this dialogue was Mantle Hood (1918–2005), an early ethnomusicologist who established a gamelan program at UCLA in the 1950s. Currently, hundreds of gamelan ensembles in the United States perform a plethora of musical styles from various islands around Indonesia. Several groups exclusively perform works composed by Americans on instruments built by Americans.

Early experiments in constructing gamelan in the United States date to the 1960s, when Dennis Murphy built a set of instruments loosely based on Javanese instruments. In the following decade, several other American-made gamelan ensembles began to crop up. They designed instruments that were also mostly inspired by Javanese rather than Balinese music. These included sets of instruments built by Daniel Schmidt, Paul Dresher, Barbara Benary, and most famously, Lou Harrison and Bill Colvig, among others. Fewer instrument-building endeavors were on the “Baliphile” side, but plenty of composers and performers created works for Balinese instruments and collaborated with groups in Bali. Three of them are Wayne Vitale, Michael Tenzer, and Evan Ziporyn. They are all still active composing for and working with Balinese music. The American Gamelan Institute (AGI), founded by Jody Diamond in 1981, researches, promotes, and publishes work related to all forms of gamelan music in Indonesia, the United States, and abroad.

Within this group of American gamelan composers, performers, instrument builders, and researchers exists a complex web of interconnections to other genres of twentieth-century American music. It reaches from American minimalism—Ziporyn has performed and premiered numerous works by Philip Glass and Steve Reich through his connection with the Bang On a Can collective—to American noise music and the downtown avant-garde—Philip Corner, who composed works for and performed on instruments built by Barbara Benary, was a part of the Fluxus group of artists and composers—to the academic contemporary/experimental music scene. (Lou Harrison was a close friend of John Cage and taught at several academic institutions, including Mills College, that fostered new American music. He also pushed for new directions in tuning and microtonality, a theme that has since caught on at AGI.)

These are only a few of the many American gamelan projects that have developed over the last century or so and predate the work at hand.

American Tuning

The model of gamelan tuning that I describe here presents one major departure from past experiments in American gamelan and other experimental American instrument-building endeavors: it is a reactive approach to tuning rather than a proactive one. The reaction in question is the incorporation of inharmonicity, which dominates the spectrum of gamelan instruments and defines their unique timbre. In this case, the tuning is designed to react to such inharmonicity as a way to incorporate it into the tuning model, rather than defend against it.

Most composers and theorists, even those who have worked substantially with inharmonic instruments, have created tuning models or compositions based on an abstract understanding of harmonic relationships. Hermann Helmholtz, the patriarch of modern-day tuning theory, addressed inharmonic resonance by saying,

Nearest to musical tones without any upper partials are those with secondary tones which are inharmonic to the prime, and such tones, therefore, in strictness, should not be reckoned as musical tones at all. They are exceptionally used in artistic music, but only when it is contrived that the prime tone should be so much more powerful than the secondary tones, that the existence of the latter may be ignored. . . . Musically, they are only available for the more or less good simple tones which they represent. . . . [Inharmonic] rods, however, are not suitable for real artistic music, although they have lately been introduced for military and dance music on account of their penetrating qualities of tone (Helmholtz 1954:70–71).

Harry Partch, who famously built an entire ensemble of instruments of his own design, based his tuning model on an extended 11-limit just intonation with 43 scale degrees to the octave, all tuned to harmonic ratios. His 36-key diamond marimba, an inharmonic idiophone, was tuned to just ratios from this 43-note scale (Partch 1974). Lou Harrison built multiple sets of aluminum American gamelan instruments, several of which were tuned to models of just intonation based on whole-number ratios derived from the harmonic series.

William Sethares, in his book Tuning, Timbre, Spectrum, Scale, posits a theory of tuning that suggests it can evolve out of the timbre of the instruments that are creating the music. He proposes Western tuning developed from prioritizing the prominent harmonic overtones when creating scales and temperaments so that the timbre of the instruments would be matched to the relationships created in their melodic and harmonic interactions. Sethares introduces the notion of sensory consonance and sensory dissonance as being related to the alignment of the overtones between resonating instruments. This refers to past work done by Helmholtz, as well as to the theory that James Tenney put forth in his publication A History of ‘Consonance’ and ‘Dissonance’ (Tenney 1988).

Sethares also posits that gamelan tuning evolved out of the inharmonic overtones within individual keys and gongs. “The musical scales of these nonwestern traditions are related to the inharmonic spectra of the instruments, and the idea of related spectra and scales is applicable cross culturally” (Sethares 2005:6). He later adds, “The inharmonic spectra of certain instruments of the gamelan are related to the unusual intervals of the pelog and slendro scales in much the same way that the harmonic spectrum of the instruments in the Western tradition is related to the Western diatonic scale” (Sethares 2005:199).

The tuning model that I describe uses this perception of tuning and timbre to build a scale that is drawn out of the spectrum of the instruments, rather than built around predetermined just or tempered intervals.

The Lightbulb Instruments: Building and Rough-tuning

In the summer of 2011, I was introduced to the sculptor Bernie Hosey at his home in Twisp, Washington, which sits in the middle of the Methow Valley at the eastern foot of the Cascades. I was invited there to take part in the Mazama Residency, where I was to be the resident sound artist. Bernie was a local artist who had gained considerable recognition in the years leading up to 2011 for his behemoth metal spheres, which were being sold for a high premium on the international market. My friend Abigail Napp, who organized the residency, thought we would work well as collaborators. Bernie was somewhat proprietary over his work, having developed his own techniques on homemade machinery in order to realize his sculptures, and he was generally protective against allowing anyone to enter his workshop and learn his techniques. Since I was a mere musician, I imagine he had little fear that I would exploit the sculptural practices he had developed, so he was willing to share his workspace with me.

Upon entering his workshop for the first time, I was taken aback by the immensity of the scale in which he worked. He had developed a cooling system by connecting a turbine to his underground well. This circulated air through the two-story cavernous barn that served as his workshop. Connected to the barn was an Aeolian harp made with three metal wires that extended two hundred yards across the valley and connected to a thirty-foot-tall sculpture designed to brace the wires. The barn functioned as a resonating chamber for the harp. As the wind swept across the valley floor, the barn converted into an ethereal echo chamber. He had also welded together his own hydraulic press. Bernie used the press, weighing over two tons, to form his metal fragmented spheres by pressing the metal between two convex and concave spherical plate inserts. He had created them in many different sizes and used the plate inserts to bend metal to precise curves.

I arrived in the Methow Valley having just finished an apprenticeship with Wayne Vitale in El Sobrante, California. Wayne taught me how to tune the instruments of a Balinese gamelan ensemble. My first thought upon seeing Bernie’s workshop was we could make gamelan keys to our own specifications by using the equipment he had. Bernie sent me to the local steelyard to buy flat bar stock. It is made to serve as reinforcement in industrial construction, but we could cut and curve the bars to various sizes in his workshop. I brought back several long bars of three different dimensions, with the idea of making keys for three different types of instruments. We had enough steel to make not only twenty keys from each of the smaller sizes of bar stock but also ten keys from the larger stock. In my mind this translated to two paired instruments, ten keys each, for both of the smaller sizes of steel bars, and one pair of five-keyed instruments made from the larger bars. This formed six instruments total: two instruments in the highest range paired to one another with ten keys each expanding across two octaves in a pentatonic scale, which I called sopranos; two instruments in the next register down, called altos, also paired to one another with ten keys each, whose top octave crossed over with the lower octave of the sopranos; and two instruments, called tenors, paired to one another with five keys each, pitched an octave below the lower octave of the altos. These become the six instruments that eventually formed the Lightbulb Ensemble (fig. 1).

To begin to develop a pentatonic scale, the first step was to cut the long bars to various lengths. Due to the space and the timeframe in which we were working, I used my intuition and musical taste to shape the pentatonic scale rather than employ predetermined calculations or pitch detection mechanisms. Much of my intuition was driven by my preference to create a pentatonic scale loosely based on the five scale degrees of pelog selisir tuning used in Balinese gong kebyar instruments, as well as by some preference, albeit secondary and subconscious, toward consonant just intervals.

We used a hydraulic horizontal band saw to cut the keys as precisely as possible to the needed lengths. I was able to make forty keys from the two smaller-sized bar stock: two sopranos and two altos made of ten keys each, with an octave crossover in the lower octave of the sopranos and the higher octave of the altos. We cut the larger bar stock into ten keys to make the tenors and found that the highest key of the tenor instruments would crossover with the lowest key from the alto instruments. Therefore, the pair of five-keyed tenors began on the second scale degree and ascended the pentatonic scale, ending on the first scale degree in unison with the lowest key of the altos.

The next step was to curve each key. I knew that manipulating the curvature of the key would affect their overtone structure in some unique way. It also would imitate the slight curve in the bronze keys created in Bali. I thought that having a uniform curve in each key, which would be very slight in short keys and more pronounced in longer keys, would produce a beautiful visual effect on the instruments. We inserted a concave and a convex plate into Bernie’s hydraulic press and used them to press each key of the ensemble. Using this procedure, the same degree of curve was pressed into each key regardless of the length of the bar. We pressed 2,000 pounds per square inch into each key so that the curve would form precisely.

Once each of the keys was curved, the next step was to rough-tune the keys to approach the final frequency desired for each key. Making cuts with the hydraulic horizontal belt saw did not provide a sufficient degree of accuracy. The rough-tuning had to be done with an angle grinder so each key could be tuned by hand. This process involved grinding away metal from the end of the key either to shorten the length or to lighten the weight on the ends of the key, thereby raising its pitch. To lower the pitch, we ground metal away from the bottom of the center of the key. This effectively weakens the fulcrum point of the key’s vibration and makes the ends heavier with respect to the center of the key, thereby lowering the pitch. Once each key was rough-tuned with the grinder, the final step was to drill holes through the nodes—the location on the bar where no vibration occurs—of the first transverse mode of the key’s vibration. This allows a string to run through the holes to suspend the keys across a frame. Upon finishing this process, I concluded my collaboration with Bernie Hosey after a week of work in August 2011.

Fine-tuning

For a variety of reasons, I was not able to fine-tune the set of keys until January 2013 in Oakland, California. Two main priorities determined the fine-tuning approach used for the full ensemble. The first was to find higher pitched keys that could be tuned to inharmonic overtones from lower pitched keys and to use this process to settle upon a precise pentatonic scale based on the rough-tuning from 2011. This was in turn the first step I took when I began fine-tuning the instruments. Once a pentatonic scale had emerged out of this process, the next priority (and the more important one) was to create variety in the beating relationships between any unison keys throughout the ensemble.

When first creating the keys, it was important that I had a general scale in mind before I began the fine-tuning. Once the keys were cut, rough-tuned, and drilled, the range in which any particular key could be tuned sharper or flatter was limited. Once the node has been located and a hole has been drilled through the key, the length can no longer be altered without potentially affecting the resonance of the key. Any alteration in the length of the key affects the relative position of the nodes. If a hole in the key does not correspond to a node, it will impede the fundamental resonance of the key.¹ There is, however, a silver lining despite these difficulties, namely, steel is a reliably stable alloy, much more so than bronze. The pitches of the keys and their overtones do not alter after they have been tuned. (Bronze keys tend to rise in pitch after the forging process.) Nevertheless, the process of tuning any given key to some overtone from another key is limited to overtones that are close in pitch to keys that have already been rough-tuned.

This limited search aimed at finding inharmonic modes from lower pitched keys that were close in pitch to the fundamental frequency of higher pitched keys. My priority was to discover a pentatonic scale in which each of the five intervals was either drawn from the fundamental frequency of a precut, rough-tuned key that also had an inharmonic mode in the scale or came from intervals that were tuned to inharmonic modes in lower pitched keys. This model is something of a departure from earlier approaches to American gamelan tuning that are based in a proactive aesthetic and design. It differs especially from the work of Lou Harrison, who tuned many of his gamelan sets to predetermined scales in just intonation. The model that I explored was intended to be reactive, so that the tuning is adjusted to the properties of the instruments and not the other way around.

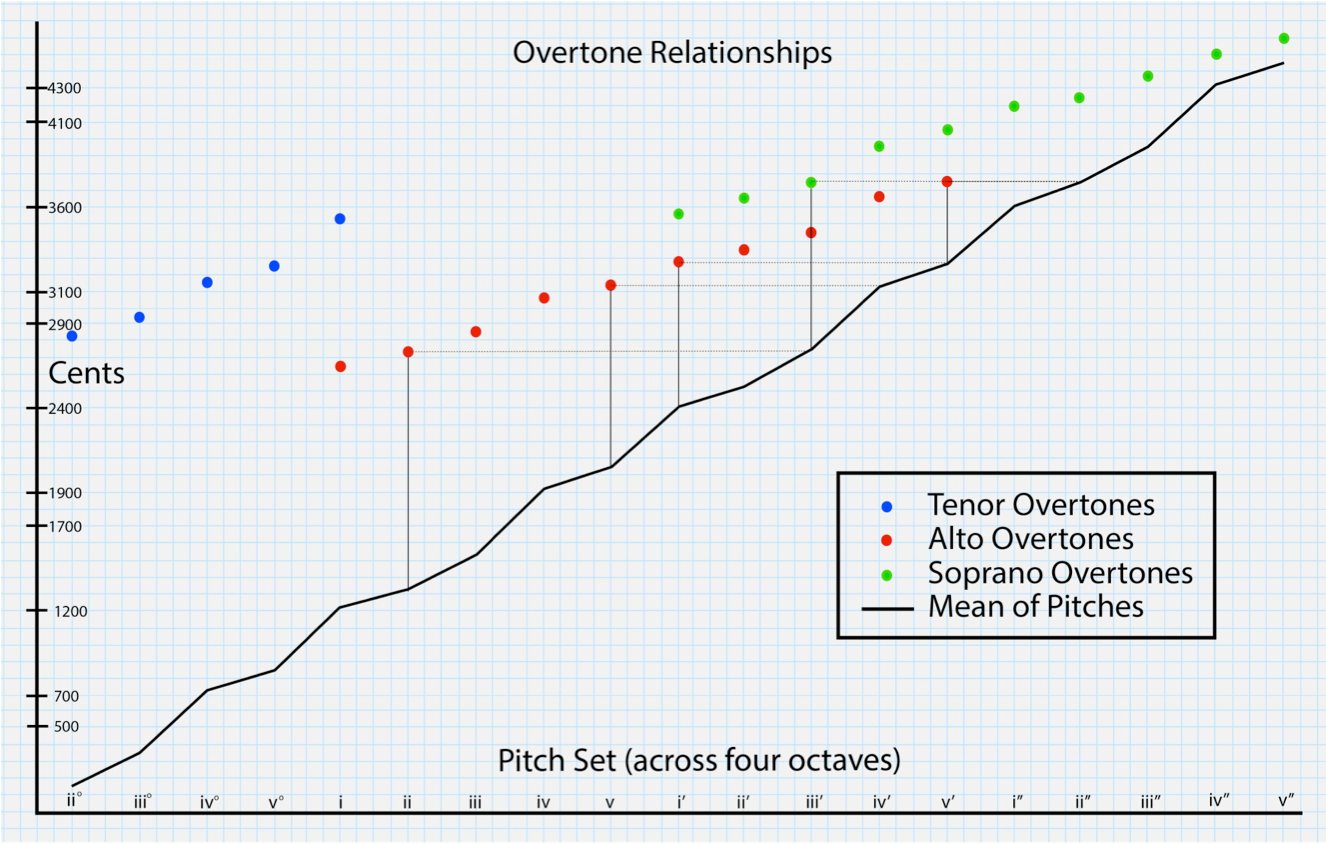
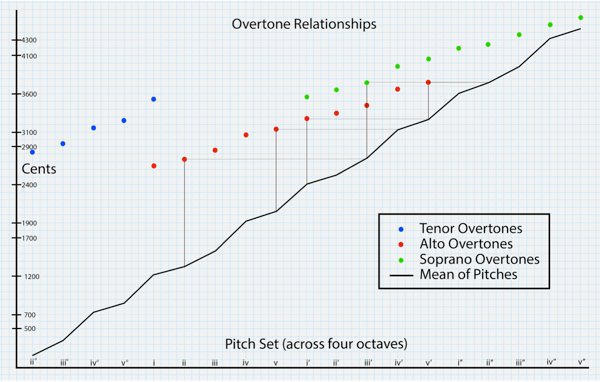
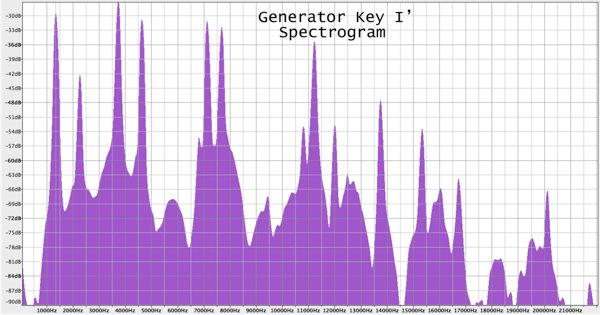
Achieving this required the use of two “generator” keys that provided inharmonic modes to create the fundamental frequencies of two higher pitched keys. The process by which this pentatonic scale was uncovered on the Alto– instrument involved several steps. (These overtones are referred to as the torsional modes in the audio example [audio 1]. A spectrogram shows the first generator key, Key I’ [fig. 2].)²

To isolate the particular inharmonic mode of vibration (in this case, the first torsional mode) on these steel keys during the tuning process, I simply dampened the center point of the key with one finger to impede the first transverse mode while I struck the edge of the key with a mallet. By doing this, I accomplished all of the tuning by ear, without using any digital frequency or spectrographic analysis.

As heard in the audio example (audio 1), the inharmonic mode in Key I’ produced the frequency by which to tune Key V’.³ In turn, Key V, tuned an octave below Key V’, had an inharmonic mode that generated the frequency for Key IV’. I had so far determined three of the five scale degrees, beginning with the fundamental frequency and torsional mode from Key I’, my first “generator” key. When I tuned Key IV an octave below Key IV’, I found the prominent inharmonic mode was not close to any of the rough cut keys, so I had to search for another “generator” key. Key II, taken from the original rough-tuning, had an inharmonic mode with a frequency to which I could tune Key III’, so Key II became my second “generator” key. I now had the five intervals of the pentatonic scale, and all the remaining keys on Alto- were tuned to octave equivalencies to this pentatonic scale. See the chart for the relationships between the scale and the overtones (fig. 3).

Several considerations to tuning octaves in gamelan instruments need to be addressed briefly. It is common in many models of gamelan tuning throughout Indonesia, and especially in Bali, to both stretch and compress the octaves across registers (Polansky 2009:72). One reason for this is to compensate for the + and - paired tuning of pengisup and pengumbang. When the ear hears two sine tones in a beating relationship, it averages the two tones and perceives the mean of the two frequencies modulating in amplitude. In Balinese gong kebyar ensembles, all of the instruments are tuned so paired instruments are approximately seven Hz apart regardless of register. The higher pitched instruments need to be tuned to an interval slightly wider than an octave above the lower pitched instruments if the two pitch averages are going to equal a 2/1 octave. This is because the ratio of the interval between the paired instruments in lower registers is larger than in higher instruments if seven Hz remains constant. At this concise mathematical representation shows, if two pengumbang keys (X- and Y-) are tuned to a 2/1 octave, then the octave between the two pengisep keys (X+ and Y+) and the mean octave between pengumbang and pengisep will not be a perfect 2/1 octave.

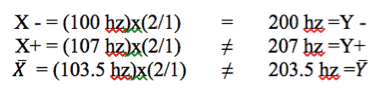
Another reason that may account for the common practice of stretching octaves in gamelan ensembles comes from a psychoacoustic phenomenon. If we were to try and tune two non-simultaneous pitches to a 2/1 octave, our tendency would be to tune the interval wide. The full explanation of that phenomenon is outside our scope here, but suffice it to say that there are several instances where our tendency is to hear octaves as being most consonant when they are slightly wide. The octave also has a different manifestation when reproduced on inharmonic instruments or electronic sequences than it does when reproduced on harmonic instruments. It is easy, and in fact more consonant, to tune harmonic instruments to perfect 2/1 octaves. If the 2/1 is slightly off, then the spectra (overtone series) of the pitches will be slightly misaligned, creating an audible dissonance. Divorced from the harmonic spectrum, as gamelan instruments are, an imperfect octave will not clash along its overtones so the spectral dissonance between the two pitches disappears. This practice of stretching octaves is also common when compensating for the slight inharmonicity throughout the full register of a piano.

Although it is common for a gamelan in Bali to stretch octaves throughout the ensemble, it is also common to tune “solo” instruments to the higher pitch of the paired tuning. This is seen especially when tuning the trompong, which spans the middle-low register of a Balinese gamelan and does not have a pair. The effect of tuning this instrument to the higher polarity helps its sound to pop out within the texture and avoid a timbral blend. The intervallic result, however, causes the instrument to add weight to the pengisup pole, raising the pitch average and thereby narrowing the octave relationship with the next register up, which sometimes ends up being squeezed instead of stretched.

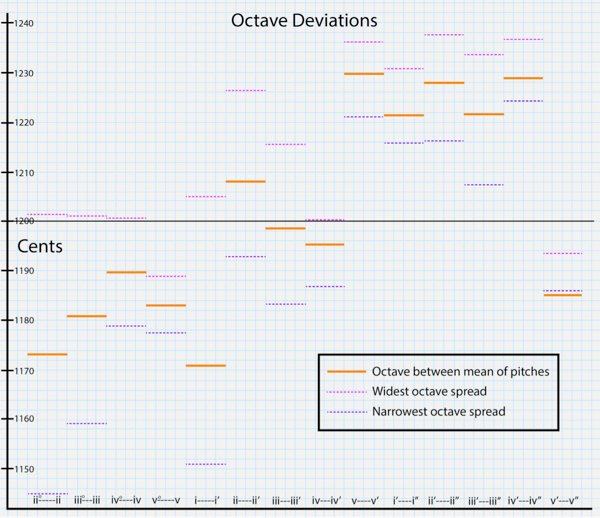
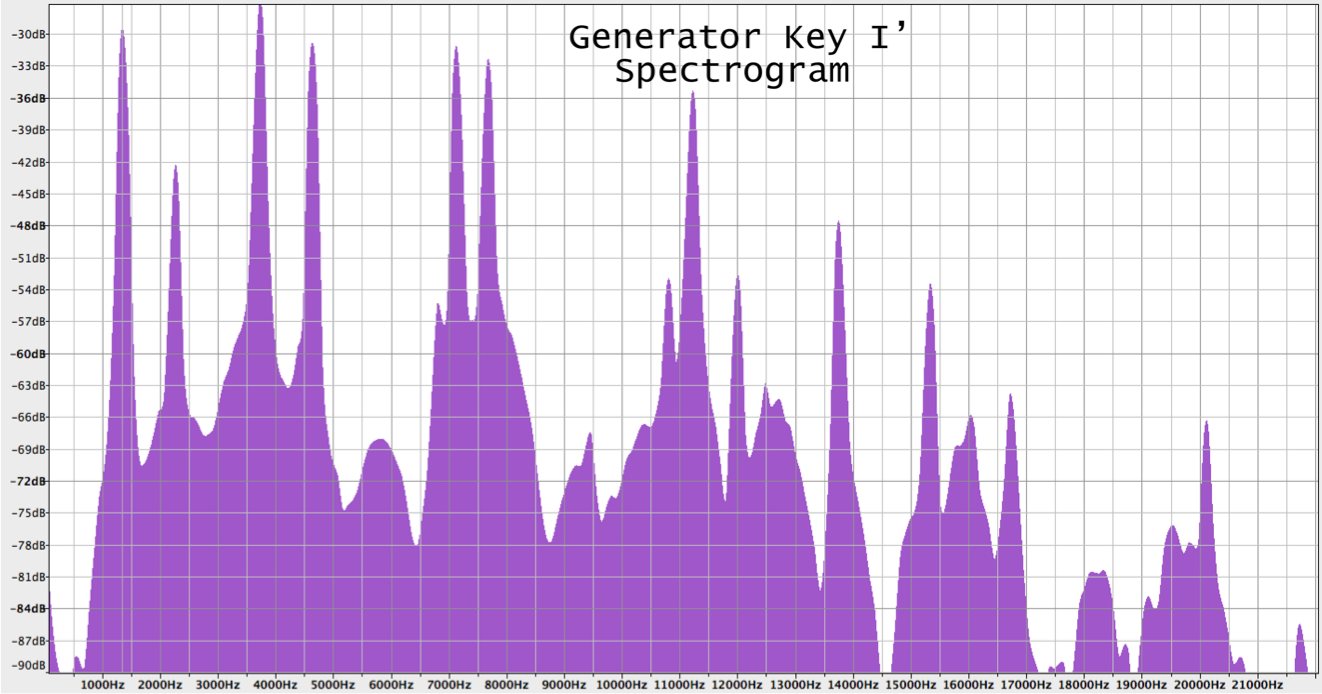
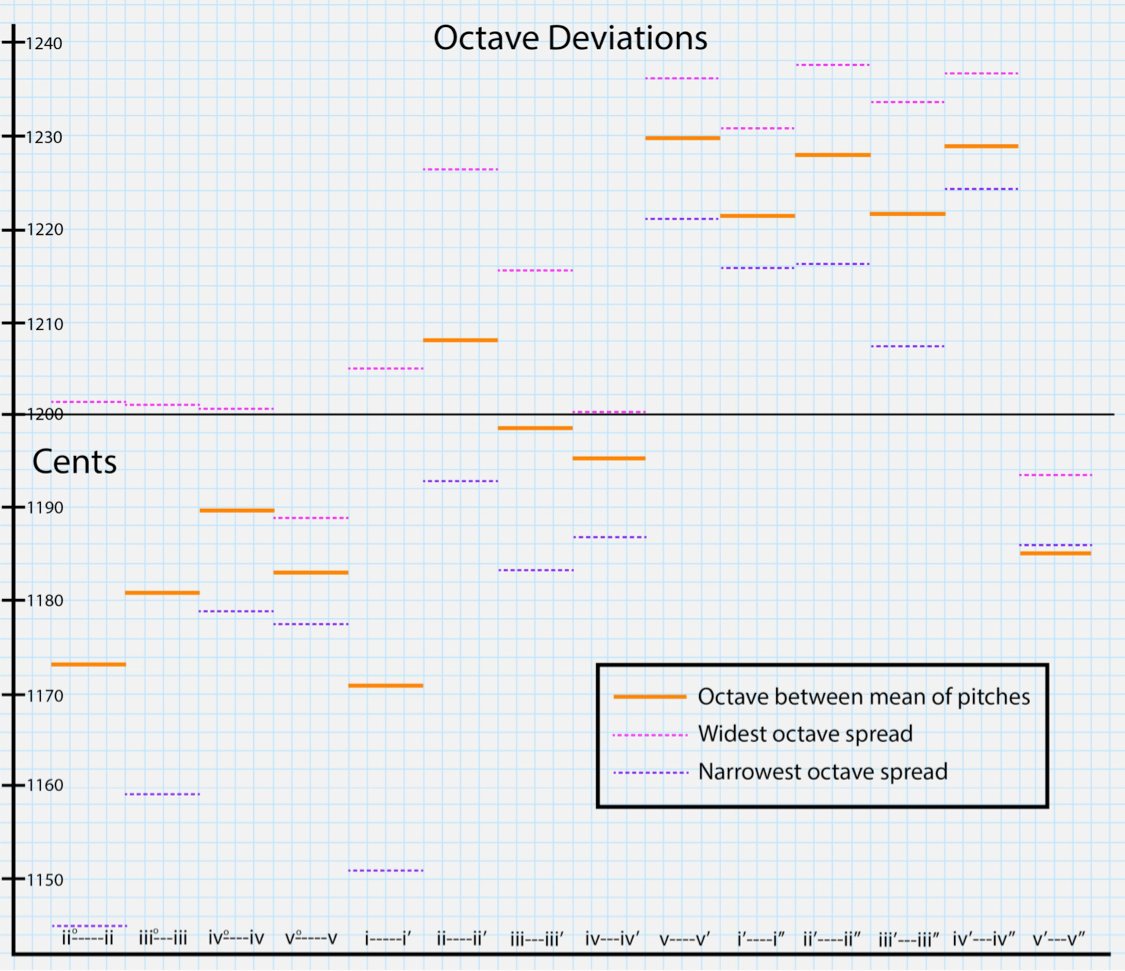
By taking an interest in these two different treatments of octaves, I decided to squeeze the octaves in the lower register, effectively making the X ̅ of the tenor instruments approximate to true octaves with the + instruments in the register above. I also stretched the upper register, effectively making the X ̅ of the soprano instruments approximate to true octaves with the + instruments in the register below. My assumption was that the tenor instruments would pop out of the texture to compensate for their lower dynamic. Above all, the progression of the octave treatment, moving from squeezed to stretched, was in line with my overall concept of symmetry for the tuning model. As seen and heard here (fig. 4 and audio 2), the deviations from a 2/1 octave, which are represented by the horizontal black line at 1200 cents, indicate the motion from squeezed to stretched octaves across the register of the instruments.⁴

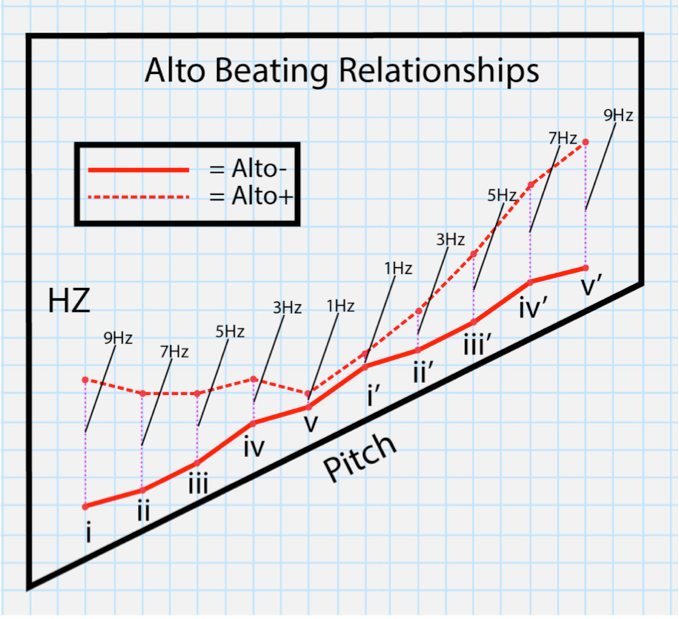
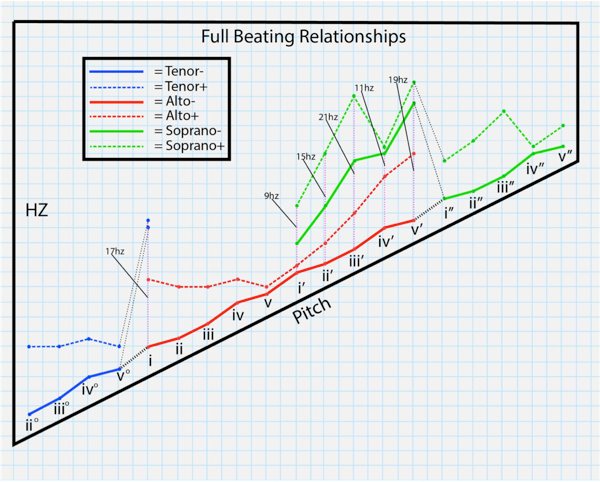
Once the intervals of the pentatonic scale were set and a general treatment of the octaves were determined, my next priority was to devise a model for variation between the beating relationships in paired instruments. The Alto– instrument was the first one to be fine-tuned, so the next instrument to tune was its pair, Alto+.

Beating Relationships/Symmetry

While thinking of a way to model the treatment of the beating relationships, several different influences guided my final decisions. The common practice in Bali is to tune entire gamelans to a unified rate of ombak, so that the beating is uniform throughout the ensemble.⁵ It is possible to squeeze or stretch the octaves across registers, but it is most always the case in Bali to keep a fixed rate of beating between pengisup and pengumbang in all octaves. The rate of beating changes, however, based on the style of music and the type of gamelan being tuned. In general, gong kebyar gamelans are tuned with an ombak around seven Hz; gender wayang instruments are often tuned slower, with an ombak around five Hz; and angklung ensembles are tuned a bit faster, with an ombak around eight or even nine Hz. Nine Hz is the upper limit used in Bali. Since the rate of ombak is always kept constant, nine Hz becomes a substantial interval in the lower octaves. For example, the intervallic distance between 110 Hz (A2) and 119 Hz is 131.1 cents, which is substantially wider than an equal-tempered semitone. The intervallic distance between 880 Hz (A5) and 889 Hz, which constitutes the same rate of beating but transposed three octaves up, is only 17.6 cents, less than one-fifth of an equal-tempered semitone.

I decided to restrict my beating model to distances between nine Hz and one Hz between any adjacent unison. Drawing influence from the tuning used by the Balinese composer Dewa Alit for his group Gamelan Salukat, I wanted variation in the beating rates throughout the ensemble in order to exploit that variation compositionally. The sizes of the tone-beats needed to be different by a measure of at least two Hz in order for their difference to be discernable. It occurred to me that I could tune the beating between Alto– and Alto+ as a palindrome by using only the odd integers between one and nine and assigning one integer to each unison in the pentatonic scale, then reversing the order in the second octave of the instruments. Therefore, the rate of beating along the ten unisons on the Alto instruments looked like this:

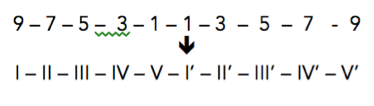
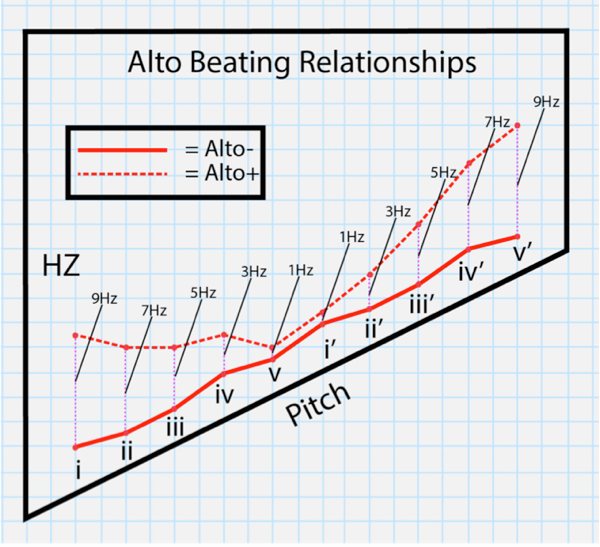
This design had several nice features. On the one hand, the symmetry of the model allows relationships to be easily conceptualized. At any given moment during the compositional process, I could remember what the rate of beating was at a particular register of the instrument set without memorizing an irregular order of beating relationships. Also, the palindrome shape creates a nice blend of similarity and difference between related pitches. Different scale degrees now became related by sharing a rate of beating. For example, pitches II and IV could be played in either octave and share a beating relationship of either seven or three Hz. Similarly, a single scale degree would now have a different rate of beating depending on which octave it was played, with the exception of scale degree III, which creates a pivot point. Finally, all of these relationships were easy to tune by ear (fig. 5 and audio 3). Again, the benefit of working with steel became apparent over time in this part of the tuning model, since these rates of beating have remained consistent years after the fine-tuning was actualized.

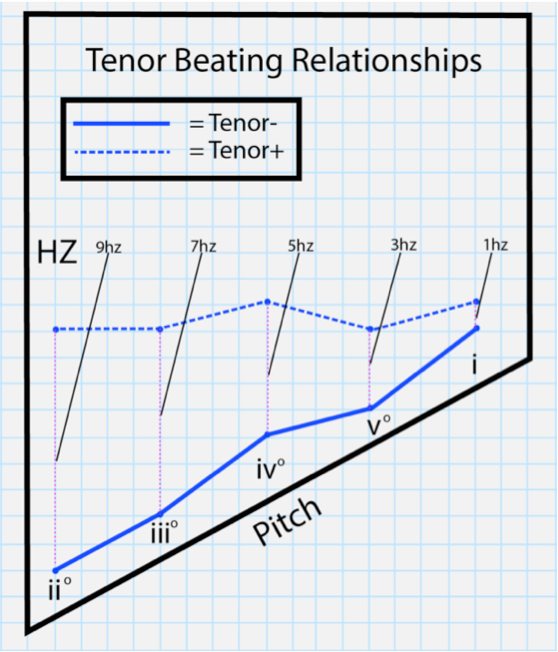
The model for the fine-tuning of the Tenor instruments was designed to be an extension of the palindrome created between the Altos, but it dovetailed at the point in which the two instruments align. The beating pattern between Tenor– and Tenor+ became:

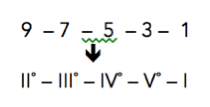
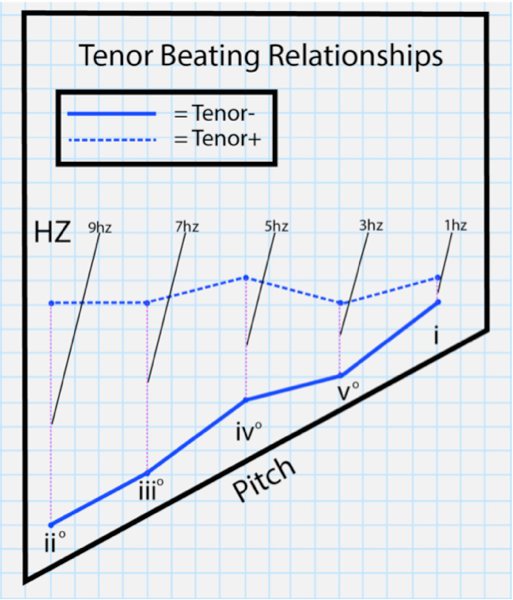
Here, I wanted to place the highest rates of beating on the lowest pitches of the ensemble to accentuate the sonic pressure produced by the beating and effectively amplify the instruments, which have a smaller dynamic range. This widening relationship also helped to squeeze the octaves between the Altos when moving down the scale of the Tenors. In addition, having a wide interval at the bottom of the register allows for different characters or interpretations of the pentatonic scale to become apparent, depending on which of the two unisons is accentuated. It creates a sort of wavering affect that ripples through the higher pitched instruments. This interval at the bottom of the ensemble, formed by the nine Hz distance between unisons on pitch II˚, is substantial, almost forty cents wide. (Refer to fig. 6 and audio 4 for the beating relationships between Tenor– and Tenor+.)

At the unique point where the Tenors and Altos align on pitch, I had an opportunity to highlight the beating cluster between the four unison keys. In keeping with the model of squeezing the octaves in that register, the Tenor pitches at scale degree were tuned sharp from Alto+. This made the relationship between Alto– and Tenor+ the largest unison separation in the whole ensemble. The distance at that point is seventeen Hz, which creates an interval of 43.2 cents and approaches the outer limit for maintaining a scale degree identity between these two unison pitches. The four pitches have markedly different characters and can be presented either as enharmonic unities or as distinct intervals.

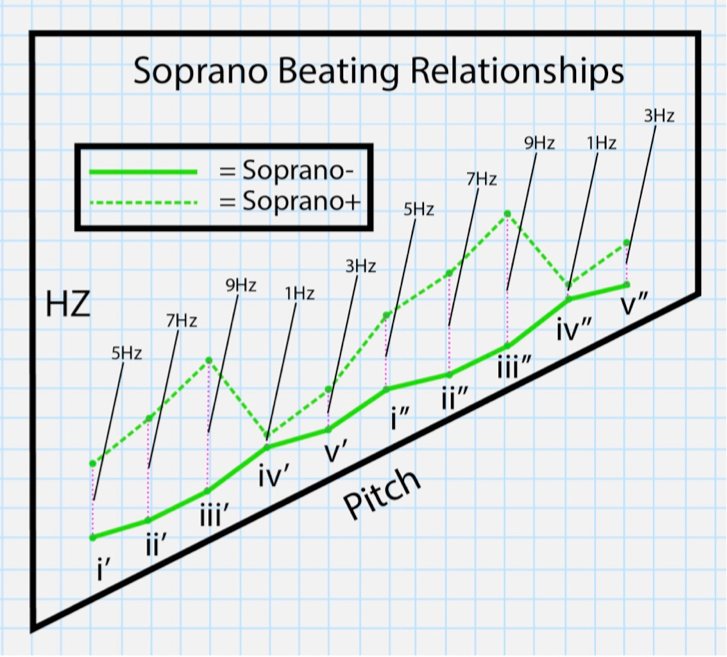
Now that I had created a model for the Alto and Tenor instruments, the last in line were the Soprano instruments. In keeping with the desire to produce a variation between all unison beatings, I created a different progression for the beating relationships in the Soprano instruments. I decided to continue to use the same odd-numbered integers for each relationship, but this time I created a progression that had a linear, periodic repetition, so that the beating relationship in both octaves was the same. The relationships became:

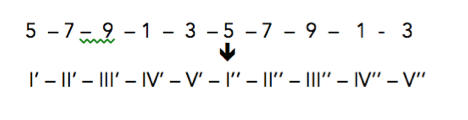
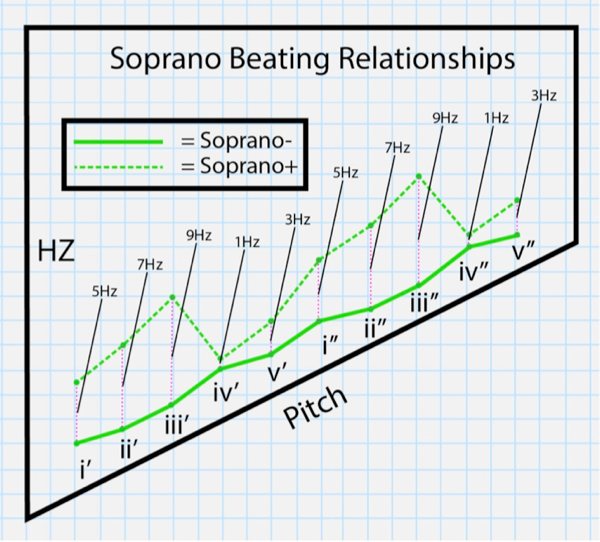
My desire for a repeating pattern in the Sopranos involved wanting to maintain a clear conceptualization of the beating relationships while creating a pattern that contrasted with the beating pattern in the Alto instruments. In the Soprano instruments, the octave relationships became the point of similarity along the beating variations. The choice to start the ascending odd-integer pattern at five Hz provided a variation with the Alto instruments, where the same unisons can be heard but a different rate of accelerating beating is discernable. That is to say, the instruments could now play an ascending scale on the Altos along pitches I’, II’, III’, etc. and produce an ascending beating pattern of one, three, five, etc., whereas if the same unisons were heard on the Sopranos, the beating would be five, seven, nine, and so on. Starting the Soprano beating pattern at five Hz also led to the highest two unisons in the ensemble being separated by one Hz and three Hz. Any larger degree of separation at pitches that high in register would be difficult to tune and also difficult to hear. (Refer to fig. 7 and audio 5 for the beating between Soprano– and Sorano+.)

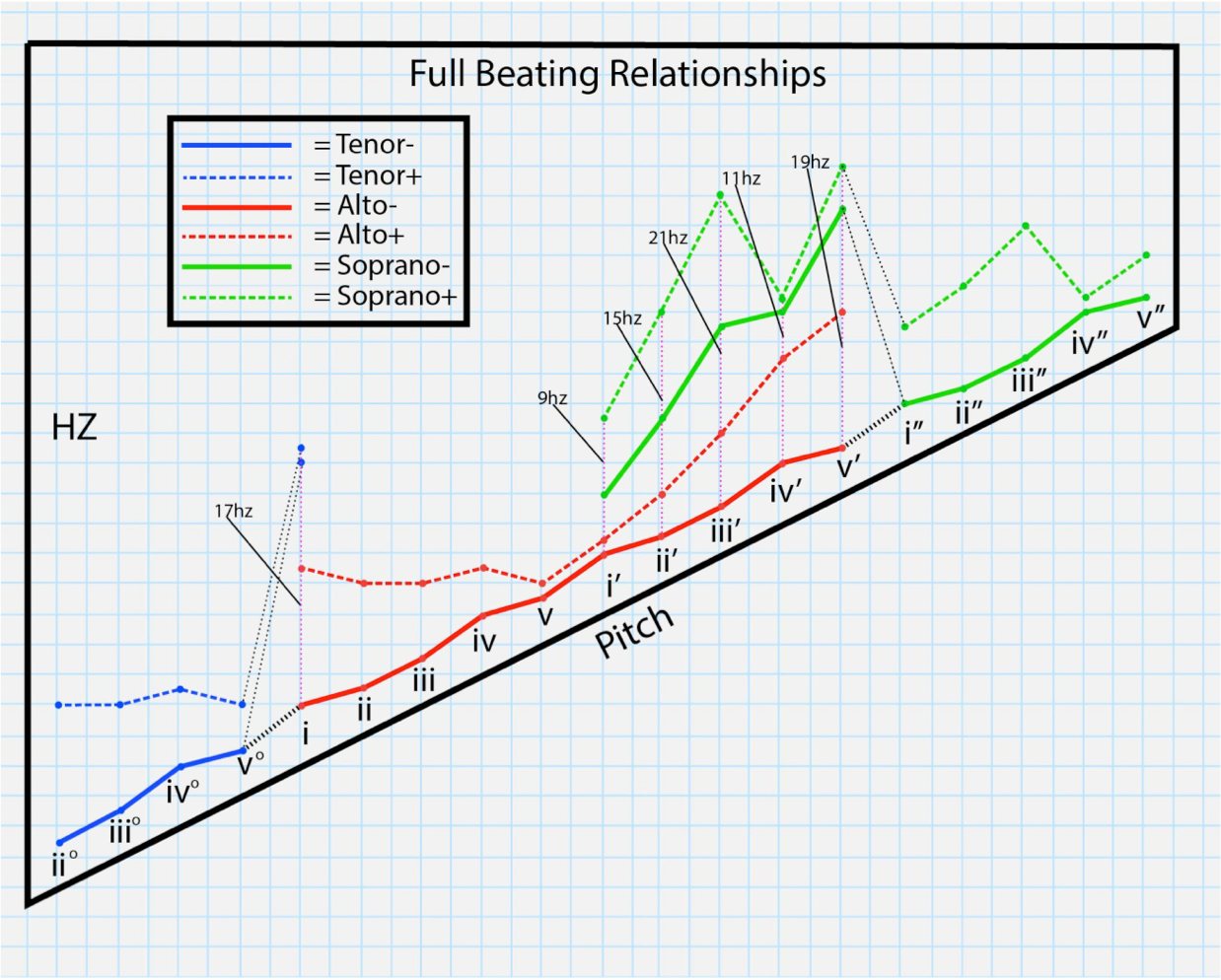
At this point, the issue of overlap between the Sopranos and Altos became an important element in the tuning model. Both instruments have pitches at I’, II’, III’, IV’, and V’. An octave overlap occurs where there would be four unison keys at each scale degree, and beat clusters form within those unisons. I had already designed the rate of beating for both the Alto and the Soprano pairs, so I needed to create a beating model between the two pairs to represent the overall cluster. The clearest approach was to keep Alto– as the lowest in frequency of the four unisons and to build up adjacencies above it. Therefore, the order in ascending frequency of the four instruments became:

Since the individual relationships within the Altos and the Sopranos had already been determined, I had to decide the beating relationship between Alto+ and Soprano-. Here, my priority was to maximize the clusters, or the total difference in Hz between Alto– and Soprano+, without challenging the integrity of the scale degree identity within unison pitches. I also needed to make these total sizes in Hz different at each scale degree in that octave. My preference was to continue to use a sequence of odd-number integers between one and nine between Alto+ and Soprano- while increasing the total size between Alto– and Soprano+. By adding the distance between Alto– and Alto+ to the distance between Soprano– and Soprano+, the initial sizes of the clusters at each scale degree were:

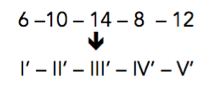
At the register where these unisons were located, the frequency distances in Hz between pitches was approximately equal to their size in cents, which made for some convenient math. By restricting the distances between unisons in Alto+ and Soprano– to nine Hz, none of the total distances were larger than twenty-three Hz. This kept the total interval size to less than one-fourth of an equal-tempered semitone, small enough so that all the unison pitches would maintain the same scale degree identity. I chose to separate Alto+ and Soprano– by this sequence of Hz deviations:

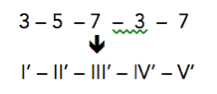
Now, the total distances in Hz between Alto– and Soprano+ were:

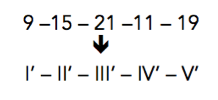
This irregular sequence of the beat clusters along this octave is meant to provide a contrast in symmetry from the micro-tuning relationships, the sum of which created this macro-sequence of clusters. The order of this sequence is significant, however, in that it mimics a moment of symmetry operation (similar to the circle-of-fifths model used for harmonic instruments). The first piece that I composed for this set of instruments was designed to exploit as well as refer to the tuning model on various levels. For example, the opening of the piece presents an extremely sparse soundscape that isolates various beating relationships between pairs and sets of instruments. The five beat clusters were used in developing the overall form of the piece. Each one of these clusters was used to represent a section of music in which the length of the metric cycle, or gong cycle, corresponds to the number associated with the total beat cluster. In doing this, I applied the same proportions to two different parameters of the whole creation—the beating relationships in the instruments and the metric divisions of the composition—which otherwise would be unrelated. Similarly, the scale degree associated with each beat cluster became the central pitch (or tonic) in the particular section of music that was derived from the beat cluster.

In keeping with the overall concept of symmetry/asymmetry in the music, all of the sections, subsections, and phrase lengths of the piece were proportioned as different sized palindromes, inspired by the palindrome created through the beating relationship between the paired Altos. These various interpretations of symmetry are¬ meant to fulfill my desire to create a multilayered complexity in the overall tuning model for the instruments as a way to exploit and refer to that complexity, like a “metaphor,” during the process of composing for the instruments. (Refer to fig. 8 and audio 6 for the beating relationships throughout the whole ensemble of instruments.)

Future Directions

In the year after finishing these instruments, I expanded the instrumentarium to include four new xylophones (wood-keyed instruments made of thuja plicata, western red cedar) that occupy the lower register of the ensemble. Since building with wood is much more workable than metal, I found it easy to expand on some of the tuning concepts I had used on the metal keys. I tuned multiple modes of vibration, or overtones (as is done with concert marimbas), to match the overall spectrum of the ensemble. With the addition of these new instruments, the ensemble has since felt somewhat complete in range and timbre, with roughly a six-octave range and a timbral variety from the combination of wood and metal keys.

This ensemble of instruments derived from models of inharmonicity does seem to have a unique overall sound quality when compared to ensembles of harmonic instruments. Although limited in the content of pitch classes, the microtonal and spectral complexities of the instruments seem to engage the ear’s attention in the higher spectrum and vertical orientation as well as in the shorter temporal frames of listening (perhaps within 500 milliseconds) in a way that is comparable in allure to music with larger pitch class sets created by harmonic instruments. Several music pieces that use Western instruments have been added to the Lightbulb instruments, including a large-scale work that incorporates a string quartet. The timbral blend between the two ensembles is fascinatingly unusual, to these ears at least. String ensembles have a capacity to sustain notes that contributes a different envelope to the sound of the gamelan. Adding strings to the instrumentarium could be an idiomatic advance in creating a fully versatile ensemble of instruments and accompanying repertoire.

In future building projects, I hope to create a new set of metal keys that incorporates some of the methods that I used in designing wooden keys, namely, tuning multiple modes of vibration to specific spectral relationships that relate to the scale used in the key set. While this would be similar to tuning concert vibraphones, my preference would be to tune the “intra-key” secondary vibrational modes to the “inter-key” scalar relationships based on spectral inharmonicities, whereas secondary modes in vibraphones are tuned to imitate harmonic overtones. Hopefully, with the addition of new instruments and new repertoire, I can continue to contribute to the ongoing phenomenon that is American gamelan.

Notes

• 1. It is also important to note that if a hole is drilled through the key at one of the nodes of the first transverse mode, it will affect the resonance of any mode that does not have nodes at that position. To search for resonant modes other than the first transverse mode, the holes must already be drilled into the key in order for those modes to be set in their frequency. Therefore, tuning the fundamental frequency of keys with holes in them to inharmonic modes from other keys with holes in them is, to some degree, inherently limited to finding frequencies that are already relatively close.
• 2. Here, the values of the first two prominent modes of vibration are 1352 Hz and 2270 Hz.
• 3. The prominent inharmonic mode produced by these keys was the torsional mode, in part because of the substantial curve introduced into the key. The torsional mode is the vibration that occurs across the diagonal axes that run the length of the key.
• 4. Notice that the several instances where the octaves are closest to a 2/1 octave occur at the widest octave between the registers. Another point of interest is the distance between the narrowest octave and the widest octave at any given scale degree, which aligns with the size of the beat clusters along those scale degrees. For example, the widest spread between the octaves occurs from I⇒I’, at the point where the Tenors and the Altos overlap.
• 5. The exception to this rule, seen in the tuning of Dewa Alit’s Gamelan Salukat, encourages each individual instrument to have a unique role in the chamber ensemble. The tuning supports such an aesthetic, allowing for complex textures to emerge through the simple interplay of two instruments. This evolution in tuning also champions the way for a new language in contemporary gamelan music, promoting more of a chamber-like aesthetic in the interrelationships between pairs of instruments.